
CBSE Class 10 Maths Formulas 2022 For Term 1 & Term 2: You’ve probably heard from your parents, teachers, and seniors about the importance of CBSE Class 10. There is no denying that this class is important in establishing your career path as well as setting the foundation for it. Math is widely regarded as the most difficult subject in Class 10. This article will serve as a one-stop destination for all of the important CBSE Class 10 Math Formulas to assist you in solving problems more quickly. So sit back, chill, and read all the way to the end!
Important CBSE Class 10 Maths Formulas 2022 For Term 1 & Term 2
Students who are appearing for upcoming Class 10 Board Exams can go through the CBSE Class 10 Maths Formulas as listed below.
CBSE Class 10 Maths Formulas (Real Numbers)
Euclid’s Division Algorithm (lemma): According to Euclid’s Division Lemma if we have two positive integers a and b, then there exist unique integers q and r such that a = bq + r, where 0 ≤ r ≤ b. (Here, a = dividend, b = divisor, q = quotient and r = remainder.)
Here you can check the important formulas related to Class 10 Maths Real Numbers.
| S. No | Type of Numbers | Description |
| 1 | Natural Numbers | N = {1,2,3,4,5 > It is the counting numbers |
| 2 | Whole number | W= {0,1,2,3,4,5> It is the counting numbers + zero |
| 3 | Integers | All whole numbers including Negative number + Positive number ……-4,-3,-2,-1,0,1,2,3,4,5… so on. Like whole numbers, integers don’t include fractions or decimals. |
| 4 | Positive integers | Z+ = 1,2,3,4,5, …… |
| 5 | Negative integers | Z– = -1,-2,-3,-4,-5, …… |
| 6 | Rational Number | A number is called rational if it can be expressed in the form p/q where p and q are integers (q> 0). Ex: P/q, 4/5 |
| 7 | Irrational Number | A number is called rational if it cannot be expressed in the form p/q where p and q are integers (q> 0). Ex: √2, Pi, … etc |
| 8 | Real Numbers | A real number is a number that can be found on the number line. Real Numbers are the numbers that we normally use and apply in real-world applications. Real Numbers include Natural Numbers, Whole Numbers, Integers, Fractions, Rational Numbers and Irrational Numbers |
HCF (Highest common factor)
HCF of two positive integers can be find using Euclid’s Division Lemma algorithm
We know that for any two integers a. b. we can write the following expression
- a=bq + r , 0≤r<b
If r=0 .then
HCF( a. b) =b
If r≠0 , then
HCF ( a. b) = HCF ( b.r)
Again expressing the integer b.r in Euclid’s Division Lemma, we get
b=pr + r1
HCF ( b,r)=HCF (r,r1)
Similarly, successive Euclid’s division can be written until we get the remainder zero, the divisor at that point is called the HCF of the a and b
- HCF (a,b) =1 – Then a and b are co primes.
- Product of primes Theorem of Arithmetic – Composite Number = Product of Primes
- HCF and LCM by prime factorization method:
HCF = Product of the smallest power of each common factor in the numbers
LCM = Product of the greatest power of each prime factor involved in the number - Important Formula HCF (a,b) X LCM (a,b) =a X b
- Important concept for rational Number – Terminating decimal expression can be written in the form p/2n5m
CBSE Class 10 Maths Formulas (Polynomials)
- (a + b)2= a2 + 2ab + b2
- (a – b)2 = a2– 2ab + b2
- a2– b2 = (a + b) (a – b)
- (a + b)3 = a3+ b3 + 3ab(a + b)
- (a – b)3 = a3– b3 – 3ab(a – b)
- a3+ b3 = (a + b) (a2 – ab + b2)
- a3– b3 = (a – b) (a2 + ab + b2)
- a4– b4 = (a2)2 – (b2)2 = (a2 + b2) (a2 – b2) = (a2 + b2) (a + b) (a – b)
- (a + b + c)2 = a2 + b2 + c2 + 2ab + 2bc + 2ac
- (a + b – c)2 = a2 + b2 + c2 + 2ab – 2bc – 2ca
- (a – b + c)2 = a2+ b2 + c2 – 2ab – 2bc + 2ca
- (a – b – c)2 = a2+ b2 + c2 – 2ab + 2bc – 2ca
- a3+ b3 + c3 – 3abc = (a + b + c)(a2 + b2 + c2 – ab – bc – ca)
CBSE Class 10 Maths Linear Equations in Two Variables Formulas
For the pair of linear equations a1 + b1y + c1 = 0 and a2 + b2y + c2 = 0, the nature of roots (zeroes) or solutions is determined as follows:
- If a1/a2 ≠ b1/b2 then we get a unique solution and the pair of linear equations in two variables are consistent. Here, the graph consists of two intersecting lines.
- If a1/a2 ≠ b1/b2 ≠ c1/c2, then there exists no solution and the pair of linear equations in two variables are said to be inconsistent. Here, the graph consists of parallel lines.
- If a1/a2 = b1/b2 = c1/c2, then there exist infinitely many solutions and the pair of lines are coincident and therefore, dependent and consistent. Here, the graph consists of coincident lines.
CBSE Class 10 Maths Quadratic Equation Formulas
For a quadratic equation, ax2 + bx + c = 0
ax2+bx+c=0 where a ≠ 0 And x = [-b ± √(b2 – 4ac)]/2a
- Sum of roots = –b/a
- Product of roots = c/a
- If roots of a quadratic equation are given, then the quadratic equation can be represented as:
x2 – (sum of the roots)x + product of the roots = 0
- If Discriminant > 0, then the roots of the quadratic equation are real and unequal/unique.
- If Discriminant = 0, then the roots of the quadratic equation are real and equal.
- If Discriminant < 0, then the roots of the quadratic equation are imaginary (not real).
CBSE Class 10 Maths Arithmetic Progression Formulas
- nth Term of an Arithmetic Progression: For a given AP, where a is the first term, d is a common difference, n is the number of terms, its nth term (an) is given as
an = a + (n−1)×d
- Sum of First n Terms of an Arithmetic Progression, Sn is given as:
Sn = n/2 [a + (n-1) d]
CBSE Class 10 Maths The similarity of Triangles Formulas
- If two triangles are similar then the ratio of their sides is equal.
![]()
- Theorem on the area of similar triangles: If two triangles are similar, then the ratio of the area of both triangles is proportional to the square of the ratio of their corresponding sides.
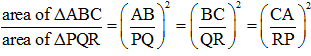
CBSE Class 10 Maths Coordinate Geometry Formulas
- Distance Formulae: Consider a line having two-point A(x1, y1) and B(x2, y2), then the distance of these points is given as:
AB= √[(x2 − x1)2 + (y2 − y1)2]
- Section Formula: If a point p divides a line AB with coordinates A(x1, y1) and B(x2, y2), in ratio m:n, then the coordinates of the point p are given as:
P={[(mx2+nx1)/(m+n)],[(my2+ny1)/(m+n)]}
- Midpoint Formula: The coordinates of the mid-point of a line AB with coordinates A(x1, y1) and B(x2, y2), are given as:
P={(x1+x2)/2,(y1+y2)/2}
- Area of a Triangle: Consider the triangle formed by the points A(x1, y1) and B(x2, y2) and C(x3, y3) then the area of a triangle is given as-
∆ABC=½ |x1(y2−y3)+x2(y3–y1)+x3(y1–y2)|
CBSE Class 10 Maths Trigonometry Formulas
In a right-angled triangle, the Pythagoras theorem states
(perpendicular )2 + ( base )2 = ( hypotenuse )2
Important trigonometric properties: (with P = perpendicular, B = base and H = hypotenuse)
- SinA = P / H
- CosA = B / H
- TanA = P / B
- CotA = B / P
- CosecA = H / P
- SecA = H/B
Trigonometric Identities:
- sin2A + cos2A=1
- tan2A +1 = sec2A
- cot2A + 1= cosec2A
Relations between trigonometric identities are given below:
- Secθ = 1/cosθ
- Cotθ = 1/tanθ
- Cosecθ = 1/sinθ
- Tanθ = Sinθ/Cosθ
Trigonometric Ratios of Complementary Angles are given as follows:
- sin (90° – A) = cos A
- cos (90° – A) = sin A
- tan (90° – A) = cot A
- cot (90° – A) = tan A
- sec (90° – A) = cosec A
- cosec (90° – A) = sec A
Values of Trigonometric Ratios of 0° and 90° are tabulated below:
| Angle | 0° | 30° | 45° | 60° | 90° |
| Sinθ | 0 | 1/2 | 1/√2 | √3/2 | 1 |
| Cosθ | 1 | √3/2 | 1/√2 | ½ | 0 |
| Tanθ | 0 | 1/√3 | 1 | √3 | Undefined |
| Cotθ | Undefined | √3 | 1 | 1/√3 | 0 |
| Secθ | 1 | 2/√3 | √2 | 2 | Undefined |
| Cosecθ | Undefined | 2 | √2 | 2/√3 | 1 |
CBSE Class 10 Maths Circles Formulas
- Circumference of the circle = 2πr
- Area of the circle = πr2
- Area of the sector of angle θ = (θ/360) × πr2
- Length of an arc of a sector of angle θ = (θ/360) × 2πr (r = radius of the circle)
CBSE Class 10 Maths Areas Related to Circles Formulas
- The equal chord of a circle is equidistant from the center.
- The perpendicular drawn from the center of a circle bisects the chord of the circle.
- The angle subtended at the center by an arc = Double the angle at any part of the circumference of the circle.
- Angles subtended by the same arc in the same segment are equal.
- To a circle, if a tangent is drawn and a chord is drawn from the point of contact, then the angle made between the chord and the tangent is equal to the angle made in the alternate segment.
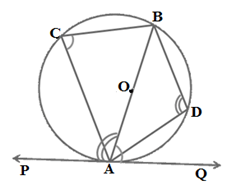
The sum of the opposite angles of a cyclic quadrilateral is always 180o.
- Area of a Segment of a Circle: If AB is a chord that divides the circle into two parts, then the bigger part is known as the major segment and the smaller one is called the minor segment.
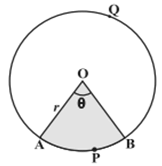
Here, Area of the segment APB = Area of the sector OAPB – Area of ∆ OAB
CBSE Class 10 Maths Surface Areas and Volumes Formulas
The common formulas from the surface area and volumes chapter in Class 10 Maths include the following:
- Sphere Formulas
| Diameter of sphere | 2r |
| Circumference of Sphere | 2 π r |
| The surface area of a sphere | 4 π r2 |
| Volume of Cylinder | 4/3 πr2 |
- Cylinder Formulas
| Circumference of Cylinder | 2 πrh |
| The curved surface area of Cylinder | 2 πr2 |
| The total surface area of Cylinder | Circumference of Cylinder + Curved surface area of Cylinder = 2 πrh + 2 πr2 |
| Volume of Cylinder | π r2 h |
- Cone Formulas
| The slant height of a cone | l = √(r2 + h2) |
| The curved surface area of a cone | πrl |
| The total surface area of a cone | πr (l + r) |
| Volume of cone | ⅓ π r2 h |
- Cuboid Formulas
| Perimeter of cuboid | 4(l + b +h) |
| Length of the longest diagonal of a cuboid | √(l2 + b2 + h2) |
| The total surface area of the cuboid | 2(l×b + b×h + l×h) |
| Volume of Cuboid | l × b × h |
Here, l = length, b = breadth and h = height In case of Cube, put l = b = h = a, as cube all its sides of equal length, to find the surface area and volumes.
CBSE Class 10 Maths Statistics Formulas
Mean: The mean value of a variable is defined as the sum of all the values of the variable divided by the number of values. ![]()
Median: The median of a set of data values is the middle value of the data set when it has been arranged in ascending order. That is, from the smallest value to the highest value.
Median is calculated as
![]()
Where n is the number of values in the data. If the number of values in the data set is even, then the median is the average of the two middle values.
Mode: Mode of statistical data is the value of that variable that has the maximum frequency
For Grouped Data:
Mean: If x1, x2, x3,……xn are observations with respective frequencies f1, f2, f3,…..fn then mean is given as:
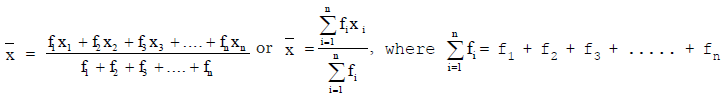
Median: For the given data, we need to have a class interval, frequency distribution, and cumulative frequency distribution. Then, the median is calculated as
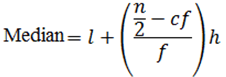
Where
l = lower limit of median class,
n = number of observations,
cf = cumulative frequency of class preceding the median class,
f = frequency of median class,
h = class size (assuming class size to be equal)
Mode: Modal class: The class interval having the highest frequency is called the modal class and Mode is obtained using the modal class.

Where
l = lower limit of the modal class,
h = size of the class interval (assuming all class sizes to be equal),
f1 = frequency of the modal class,
f0 = frequency of the class preceding the modal class,
f2 = frequency of the class succeeding in the modal class.
CBSE Class 10 Maths Probability Formulas
![]()
Download CBSE Class 10 Maths Formulas 2022 For Term 1 & Term 2
Download CBSE 10th Maths Chapter-Wise Formulas
Other Important Links Related To CBSE Class 10 Maths 2022 For Term 1 & Term 2
- Class 10 Maths Syllabus
- Class 10 Maths Sample Papers
- Class 10 Maths NCERT Solution
- Class 10 Maths RD Sharma Solutions
- Class 10 Maths Worksheets
- Class 10 Maths RS Aggarwal Solutions
- Class 10 Maths Previous Year Papers
- CBSE Class 10 Mathematics Question Bank
- Class 10 Maths Oswaal Sample Papers
- Class 10 Maths Revision Notes
We have provided the CBSE Class 10 Maths formulas according to the latest syllabus of the academic year. Go through the below-listed CBSE Class 10 Maths Formulas carefully and prepare well for the Class 10 Exams.
FAQs on CBSE Class 10 Maths Formulas 2022 For Term 1 & Term 2
From where can I download the chapter-wise CBSE Class 10 Maths Formulas PDF?
You can download CBSE Class 10 Maths Formulas PDF from the link mentioned in the above blog.
How much does it cost to download the Class 10 Maths all formulas chapter-wise?
You can download it for free.
Can I get the detailed Class 10 Maths formulas?
Yes, In the above article you can get the detailed Class 10 Maths formulas.
Is Class 10 Maths hard?
Yes, if you do not study regularly you will definitely find Class 10th Maths hard.
