
NCERT Solutions Class 12 Maths Chapter 7 Exercise 7.9: You can prepare for the upcoming CBSE Class 12 Maths exams by studying NCERT Solutions Class 12 Maths Chapter 7 Exercise 7.9. There is a step by step solutions designed for a simple and easy understanding.
To understand the NCERT Solutions of Class 12 Mathematics and excel in their Ex 7.9 Class 12 Maths skills, you can download its PDF.
NCERT Solutions Class 12 Maths Exercise 7.9 Chapter 7
NCERT Solutions for Class 12 Maths Chapter 7 Exercise 7.9 PDF
Exercise 7.9 Chapter 7 – Integrals is based on the topics mentioned below:
Fundamental Theorem of Calculus
- Area function
- The first fundamental theorem of integral calculus
- The second fundamental theorem of integral calculus
Download NCERT Solutions for Chapter 7 Exercise 7.9 Maths Class 12 PDF:
NCERT Solutions for Exercise 7.9 Maths Class 12 Free PDF
Access NCERT Solutions Class 12 Maths Chapter 7 Exercise 7.9
Question 1.
∫1−1(x+1)dx
Solution:
Question 2.
∫321xdx
Solution:
Question 3.
∫21(4×3−5×2+6x+9)dx
Solution:
Question 4.
∫π40sin2xdx
Solution: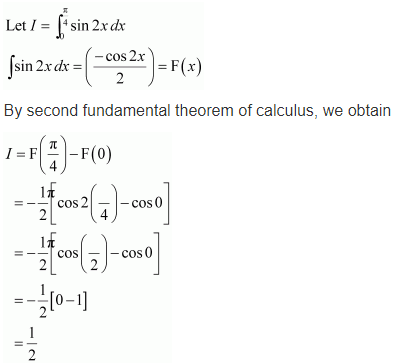
Question 5.
∫π20cos2xdx
Solution:
Question 6.
∫54exdx
Solution:
Question 7.
∫π40tanxdx
Solution: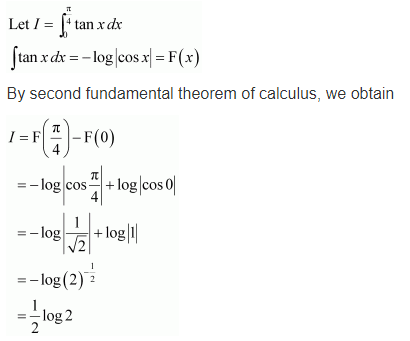
Question 8.
∫π4π6cosecxdx
Solution:
Question 9.
∫10dx1−x2√
Solution: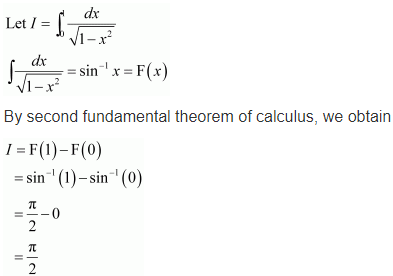
Question 10.
∫10dx1+x2
Solution:
Question 11.
∫32dxx2−1
Solution:
Question 12.
∫π20cos2xdx
Solution:
Question 13.
∫32xx2+1dx
Solution: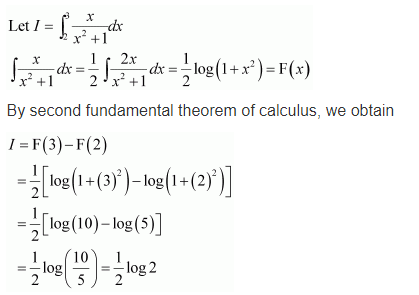
Question 14.
∫102x+35×2+1dx
Solution:
Question 15.
∫10xex2dx
Solution: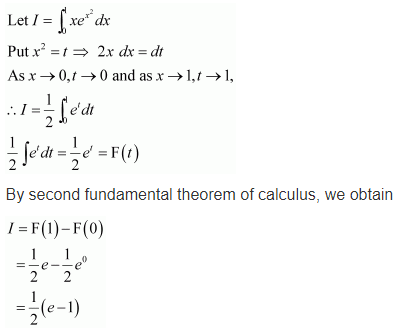
Question 16.
∫215x2x2+4x+3dx
Solution:
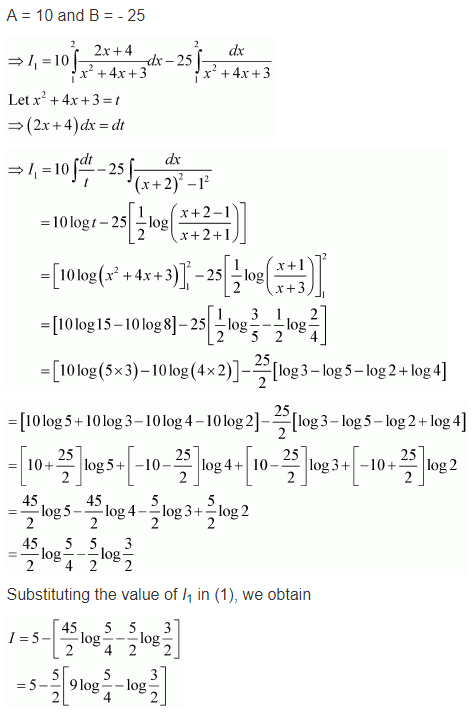
Question 17.
∫π40(2sec2x+x3+2)dx
Solution:
Question 18.
∫π0(sin2x2−cos2x2)dx
Solution:
Question 19.
∫206x+3×2+4dx
Solution:
Question 20.
∫10(xex+sinπx4)dx
Solution:
Question 21.
∫3√1dx1+x2equals
(a) π3
(b) 2π3
(c) π6
(d) π12
Solution:
Question 22.
∫230dx4+9x2equals
(a) π6
(b) π12
(c) π24
(d) π4
Solution: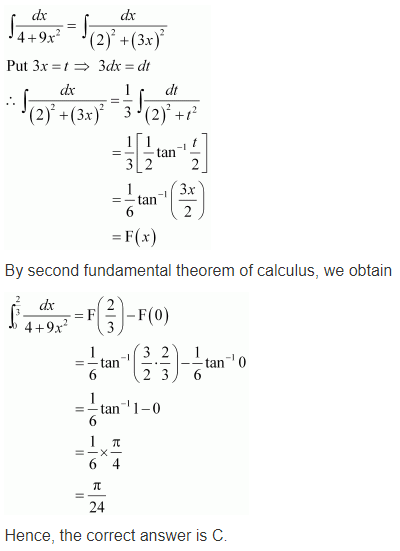
All the best to the students appearing for the Class 12th board exam. Here is the detailed blog of NCERT Solutions Class 12 Maths Chapter 7 Exercise 7.9. For further queries regarding the CBSE Class 12th exam, you can ask in the comment box.
FAQs on the NCERT Solutions for Exercise 7.9 Maths Class 12
What are the topics we can study in Class 12 Chapter 7 calculus?
Fundamental Theorem of Calculus
Area function
The first fundamental theorem of integral calculus
The second fundamental theorem of integral calculus
How much does the downloading of NCERT Solutions Class 12 Maths Exercise 7.9 PDF costs?
It is free of cost.
Are there any explanations of the solved questions?
Yes, explanations are also given along with the solutions.
From where can we download NCERT Solutions Class 12 Maths Exercise 7.9 PDF?
You can download it from Kopykitab.
Why to study from NCERT Solutions Class 12 Maths Chapter 7?
Here are some of the reasons to study from NCERT Solutions Class 12 Maths Chapter 7:
High-quality content with reliable and accurate solutions.
Subject matter experts have designed the solutions.
All the answered are solved with steps and proper explanations, for your better understanding of concepts.
The PDF form of the solutions is available which is easy to download and free of cost.
