The fourth exercise of the RD Sharma Chapter 1 Number System for Class 9th is based on representing the real numbers on the number line. In the CBSE textbook of Class 9th, a stepwise and detailed explanation is given for each question. We are provided the RD Sharma Chapter 1 Class 9 Maths Exercise 1.4 Solutions prepared by our experts by following CBSE guidelines to complete the syllabus accordingly. Doing practice on these questions helps to score well in the exams.
In this article, students get the complete detailed solutions of the Class 9th Maths based on the CBSE Syllabus. Here the explanation of the questions is based on the real numbers on the number line.
Download RD Sharma Chapter 1 Class 9 Maths Exercise 1.4 Solutions PDF
Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.4
Important Definition RD Sharma Chapter 1 Class 9 Maths Exercise 1.4 Solutions
In the following points, we have defined the real numbers on the number line with examples.
- A number can neither be signified as a terminating decimal nor as a repeating decimal is called an irrational number. For Example- 0.10100100010000…
- All rational numbers are expressible either as a terminating decimal or as a repeating decimal. For Example, rational numbers are- 6.2876.
Irrational Number on the Number Line
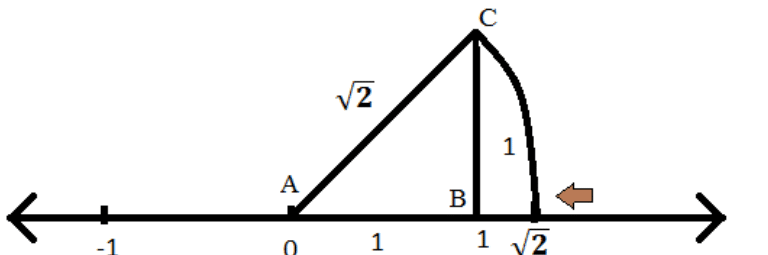
Check how the irrational number will be shown on the number line. For Example, take as an irrational number-
- Draw a number line, indicate the origin and other integers.
- Find the distance between 0 and 1 using the device.
- Draw a perpendicular to 1 of the equal length as between 0 and 1.
- Join origin and another end of the new line, i.e., perpendicular. You will get a figure as shown.
- Name origin as A, 1 on the number line as B & end of perpendicular as C.
- The range between A and C is √2.
- Use a device (compass) to find the distance between A and C.
- Place device (compass) at A and mark the equal length between A and C on the number line.
- Get the point √2 at D.
- Likewise, please do it for other irrational numbers like √3, √5.
Integers on the Number Line
- The right side number means a positive sign.
- The left side number means a negative sign.
Decimals Number on the Number Line
Like 0.5 has the whole number 0, it will lie between 0 and 1 and divide the line between 0 & 1 into 10 equal parts. Then, 0.5 will lie five parts right to the 0.
Frequently Asked Questions (FAQs) of RD Sharma Chapter 1 Class 9 Maths Exercise 1.4 Solutions
Ques)- What is the difference between the real line and the number line?
Ans)- The set of real numbers are denoted with “R.” Real Numbers are the set of the Rational Numbers, Irrational Numbers, Whole Numbers, Natural Numbers, Integers, etc.
Whereas the larger number always lies to the right side of the smaller ones in the number line. It is clear that 13 is greater than 3, but it may not be so apparent to understand that −1 is greater than −3 till we graph the number on a number line.
Ques)- How many real numbers are there between 0 and 1?
Ans)- Any number that lies between 0 and 1 is INFINITE. Moreover, the numbers between any two numbers like- between 4 and 7 is also called INFINITE.
Ques)- What is the number line rule?
Ans)- A number line is used to both positive and negative integers. Writing numbers on a number line cause analyzing numbers easier.
A number line can be used to move out addition, subtraction, and multiplication.
- Move right to add.
- Move left to subtract.
- Skip count to multiply.
