This chapter of Class 9 will help students to learn about congruent triangles. As we know, a triangle is a three-sided polygon holding three angles that add up to 180 degrees. But, when it comes to the congruence of triangles, two triangles are congruent if they overlay on each other or if the sides and angles should have the same lengths and measurements. In the RD Sharma Chapter 10 Class 9 Maths Exercise 10.1 Solutions is based on the topics like- Congruence of Line segments, Congruence of Line Angles, Congruence of Triangles, Congruence Relation, Congruence Criteria, etc.
The PDF attached below is prepared by our experts in which questions are collected from teh previous year’s question papers, CBSE Text Book, and RD Sharma of Class 9. These questions will help to understand the topics of this exercise, and the stepwise easy explanation will help learners to score well in the exam.
Learn about RD Sharma Class 9 Chapter 10- Congruent Triangles
Download RD Sharma Chapter 10 Class 9 Maths Exercise 10.1 Solutions PDF
Solutions for Class 9 Maths Chapter 10 Congruent Triangles Exercise 10.1
Important Definitions RD Sharma Chapter 10 Class 9 Maths Exercise 10.1 Solutions
Here is the list of the topics and subtopics of the Congruent Triangles-
- Congruence of Line segments
- Congruence of Angles
- Congruence of Triangles
- Congruence Relation
- Congruence Criteria
- SAS (Side-Angle-Side)
- SSS (Side-Side-Side)
- ASA (Angle-Side-Angle)
- RHS (Right angle- Hypotenuse-Side)
Congruence of Line segments
Line segments are congruent if they possess the same length. Though, they must not be parallel. Line Segments can be at any angle or adjustment on the plane.
It is possible to form (draw) a congruent line segment to a provided segment with a compass (protractor) and straightedge.
Congruence of Angles
These are the angles (with the same radians or degrees) with the same measures.
- Congruence Angles do not have to point in the equivalent direction.
- Congruence Angles do not have to be on equal-sized lines.
Congruence Criteria of Triangles
The following are the different criteria of Congruency of Triangles-
- SAS (Side-Angle-Side)- If any two sides and an angle comprised between the sides of one triangle are similar to the corresponding two sides and an angle within the sides of the second triangle, then the two triangles are assumed to be congruent by Side-Angle-Side (SAS) rule.
- SSS (Side-Side-Side)- If all the three sides of one triangle are similar to the corresponding three sides of the second triangle, the two triangles are assumed to be congruent with the Side-Side-Side (SSS) rule.
- ASA (Angle-Side-Angle)- If any two angles and the sides involved between an angle of one triangle are similar to the corresponding two angles and the side involved between angles of the second triangle, then the two triangles are assumed to be congruent by the Angle-Side-Angle (ASA) rule.
- RHS (Right angle- Hypotenuse-Side)- If the hypotenuse and the side of a right-angled triangle are similar to the hypotenuse and a side of the second right-angled triangle, then the two right triangles are assumed to be congruent by the Right angle- Hypotenuse-Side (RHS) rule.
Examples of RD Sharma Chapter 10 Class 9 Maths Exercise 10.1 Solutions
Ques: In the below figure, the sides BA and CA have been provided such that BA = AD and CA = AE. Prove the segment DE ∥ BC.
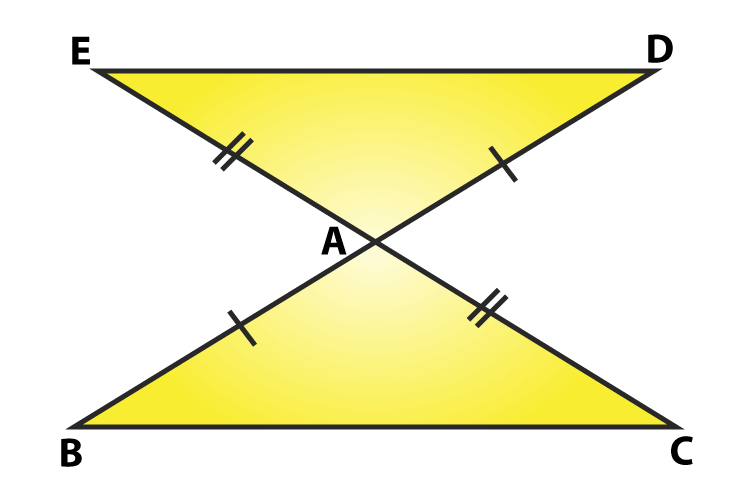
Solution-
Sides BA and CA have been provided such that BA = AD and CA = AE.
Prove- DE ∥ BC
= Consider △BAC and △DAE,
= BA = AD and CA= AE (Given)
= ∠BAC = ∠DAE (vertically opposite angles)
= By SAS congruence criterion, we have
= △ BAC ≃ △ DAE
= We know, corresponding positions of congruent triangles are similar
= So, BC = DE and ∠DEA = ∠BCA, ∠EDA = ∠CBA
= Now, DE and BC are two lines intersected by a transversal DB s.t.
= ∠DEA= ∠BCA (alternate angles are similar)
= Therefore, DE ∥ BC (Hence Proved).