In this exercise of Triangles and their angles, students will learn about the Exterior Angles of a Triangle. RD Sharma Chapter 9 Class 9 Maths Exercise 9.2 Solutions is based on the fundamental theories of exterior angles of a triangle, which says that, if a side of a triangle is given, the exterior angle so set is equal to the total of the two interior opposite angles. Go down to the article to know more about the triangle’s exterior angles. This section is one of the easiest exercises to score well in the examination.
Students are suggested to practice with the RD Sharma Chapter 9 Class 9 Maths Exercise 9.2 Solutions PDF attached to this article that helps to score well in the exam. The PDF is prepared by our experts with complete informative solutions. The PDF is available for free to do practice to score well in the exam.
Learn more about Triangles and its Angles of Chapter 9 Class 9
Download RD Sharma Chapter 9 Class 9 Maths Exercise 9.2 Solutions PDF
Solutions for Class 9 Maths Chapter 9 Triangle and its Angles Exercise 9.2
Important Definitions RD Sharma Chapter 9 Class 9 Maths Exercise 9.2 Solutions
A triangle is a three-sided structure with three interior angles. Also, there are other angles outside the triangle, which are known as exterior angles.
Here we will learn about the following points-
- The Theorem of Triangle exterior angle
- Find the unknown exterior angle of a triangle
Triangle Exterior angle Theorem
The theorem of the exterior angle declares that the sum of each exterior angle of a triangle is equal to the total of the non-adjacent and opposite interior angles.
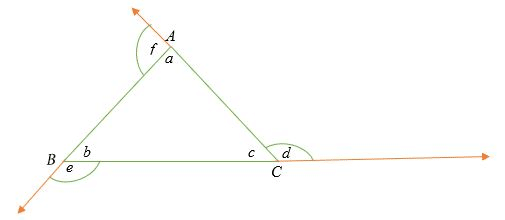
Note: The two non-adjacent interior angles opposite to the exterior angle are sometimes introduced as a remote interior angle.
For example, in the above triangle ABC-
= d = b + a
= e = a + c
= f = b + c
Properties of exterior angles
An exterior angle of a triangle is equivalent to the total of the two opposite interior angles.
The total of the exterior angle and interior angle is equivalent to 180 degrees.
= c + d = 180° (180 degrees)
= a + f = 180° (180 degrees)
= b + e = 180° (180 degrees)
All exterior angles of the triangle add up to 360° (360 degrees).
Proof:
= d + e + f = b + a + a + c + b + c
= d +e + f = 2a + 2b + 2c
= 2 (a + b + c)
According to the triangle angle sum theorem.
a + b + c = 180° (180 degrees)
Therefore, = d +e + f = 2(180°)
= 360°, Hence Proved
Get the Unknown Exterior Angle of a Triangle
The rules to find the triangle’s unknown exterior angles are the same as the rules of interior angles of a triangle. Because wherever there is an exterior angle, it exists with an interior angle, and both of them add up to 180° (180 degrees).
For Example-
The two interior angles 25° and (a + 15) ° are non-adjacent to an exterior angle (3a – 10) °, find the value of a.
Solution-
Apply the theorem of Triangle exterior angle-
= (3a − 10) = (25) + (a + 15)
= (3a − 10) = (25) + (a +15)
= 3a −10 = a + 40
= 3a – 10 = a + 40
= 3a = a + 50
= 3a = a + 50
= 2a = 50
= a = 25
Hence, a = 25°
Replace the value of x into the three equations.
= (3a − 10) = 3(25°) – 10°
= (75° – 10°) = 65°
= (a+15) = (25° + 15°) = 40°
So, the angles are 25°, 40°, and 65°.
Frequently Asked Questions (FAQs) of RD Sharma Chapter 9 Class 9 Maths Exercise 9.2 Solutions
Ques 1- What is the sum of the exterior angles of a triangle?
Ans- An exterior angle of the triangle is equivalent to the two opposite interior angles. The total of the exterior angle and interior angle is equivalent to 180 degrees.
Ques 2- Are the exterior angles of a triangle equal to 360?
Ans- The exterior angles of a triangle make a linear pair with the interior angles by increasing the triangle’s sides. The sum of the exterior angles of the triangle and any polygon is 360 degrees.
