RD Sharma Solutions Class 11 Maths Chapter 5 – Trigonometric Functions (Updated For 2021-22)

RD Sharma Solutions Class 11 Maths Chapter 5-Trigonometric Functions: RD Sharma Solutions Class 11 Maths Chapter 5 is here for you to prepare the 5th chapter (Trigonometric Functions) from your class 11 Mathematics syllabus. Chapter 5 from class 11 talks about Trigonometric Functions which is one of the Important chapters which needs clarity. And with a significant guide by side, getting thorough with the chapter might make you confident with the subject.

• Class 11 Maths RD Sharma Solutions PDF

Download RD Sharma Solutions Class 11 Maths Chapter 5 PDF

RD Sharma Solutions Class 11 Maths Chapter 5

Exercise wise: RD Sharma Solutions Class 11 Maths Chapter 5

Class 11 RD Sharma Chapter 5 Exercise 5.1

Class 11 RD Sharma Chapter 5 Exercise 5.2

Class 11 RD Sharma Chapter 5 Exercise 5.3

Access RD Sharma Solutions Class 11 Maths Chapter 5

EXERCISE 5.1 PAGE NO: 5.18

Prove the following identities:

1. sec⁴ x – sec² x = tan⁴ x + tan² x

Solution:

Let us consider LHS: sec⁴ x – sec² x

(sec² x)² – sec² x

By using the formula, sec² θ = 1 + tan² θ.

(1 + tan² x) ² – (1 + tan² x)

1 + 2tan² x + tan⁴ x – 1 – tan² x

tan⁴ x + tan² x

= RHS

∴ LHS = RHS

Hence proved.

2. sin⁶ x + cos⁶ x = 1 – 3 sin² x cos² x

Solution:

Let us consider LHS: sin⁶ x + cos⁶ x

(sin² x) ³ + (cos² x) ³

By using the formula, a³ + b³ = (a + b) (a² + b² – ab)

(sin² x + cos² x) [(sin² x) ² + (cos² x) ² – sin² x cos² x]

By using the formula, sin² x + cos² x = 1 and a² + b² = (a + b) ² – 2ab

1 × [(sin² x + cos² x) ² – 2sin² x cos² x – sin² x cos² x

1² – 3sin² x cos² x

1 – 3sin² x cos² x

= RHS

∴ LHS = RHS

Hence proved.

3. (cosec x – sin x) (sec x – cos x) (tan x + cot x) = 1

Solution:

Let us consider LHS: (cosec x – sin x) (sec x – cos x) (tan x + cot x)

By using the formulas

cosec θ = 1/sin θ;

sec θ = 1/cos θ;

tan θ = sin θ / cos θ;

cot θ = cos θ / sin θ

Now,

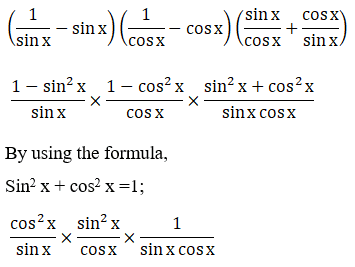
1 = RHS

∴ LHS = RHS

Hence proved.

4. cosec x (sec x – 1) – cot x (1 – cos x) = tan x – sin x

Solution:

Let us consider LHS: cosec x (sec x – 1) – cot x (1 – cos x)

By using the formulas

cosec θ = 1/sin θ;

sec θ = 1/cos θ;

tan θ = sin θ / cos θ;

cot θ = cos θ / sin θ

Now,

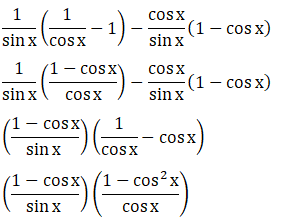
By using the formula, 1 – cos²x = sin²x;

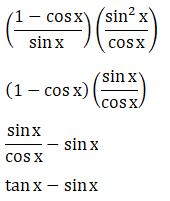
= RHS

∴ LHS = RHS

Hence Proved.

5. 

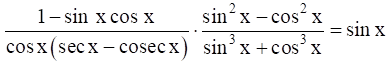
Solution:

Let us consider the LHS:

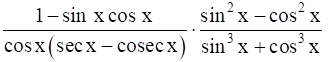
By using the formula,

cosec θ = 1/sin θ;

sec θ = 1/cos θ;

Now,

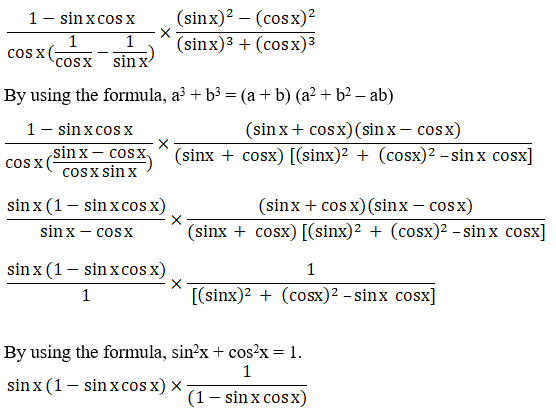
sin x

= RHS

∴ LHS = RHS

Hence Proved.

6.

Solution:

Let us consider the LHS:

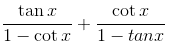
By using the formula,

tan θ = sin θ / cos θ;

cot θ = cos θ / sin θ

Now,

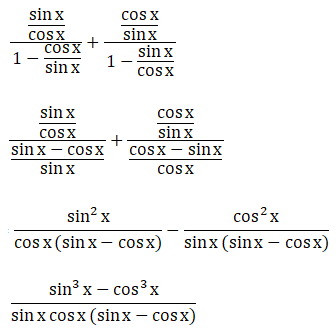
By using the formula, a³ – b³ = (a – b) (a² + b² + ab)

By using the formula,

cosec θ = 1/sin θ,

sec θ = 1/cos θ;

cosec x × sec x + 1

sec x cosec x + 1

=RHS

∴ LHS = RHS

Hence Proved.

7. 

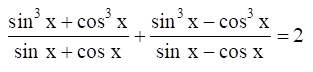
Solution:

Let us consider LHS:

By using the formula a³ ± b³ = (a ± b) (a² + b²∓ ab)

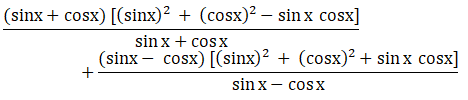
We know, sin²x + cos²x = 1.

1 – sinx cosx + 1 + sinx cosx

2

= RHS

∴ LHS = RHS

Hence Proved.

8. (sec x sec y + tan x tan y)² – (sec x tan y + tan x sec y)² = 1

Solution:

Let us consider LHS:

(sec x sec y + tan x tan y)² – (sec x tan y + tan x sec y)²

Expanding the above equation we get,

[(sec x sec y)² + (tan x tan y)² + 2 (sec x sec y) (tan x tan y)] – [(sec x tan y)² + (tan x sec y)² + 2 (sec x tan y) (tan x sec y)] [sec² x sec² y + tan² x tan² y + 2 (sec x sec y) (tan x tan y)] – [sec² x tan² y + tan² x sec² y + 2 (sec² x tan² y) (tan x sec y)]

sec² x sec² y – sec² x tan² y + tan² x tan² y – tan² x sec² y

sec² x (sec² y – tan² y) + tan² x (tan² y – sec² y)

sec² x (sec² y – tan² y) – tan² x (sec² y – tan² y)

We know, sec² x – tan² x = 1.

sec² x × 1 – tan² x × 1

sec² x – tan² x

1 = RHS

∴ LHS = RHS

Hence proved.

9.

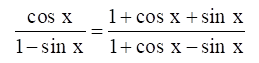
Solution:

Let us Consider RHS:

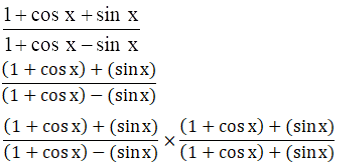
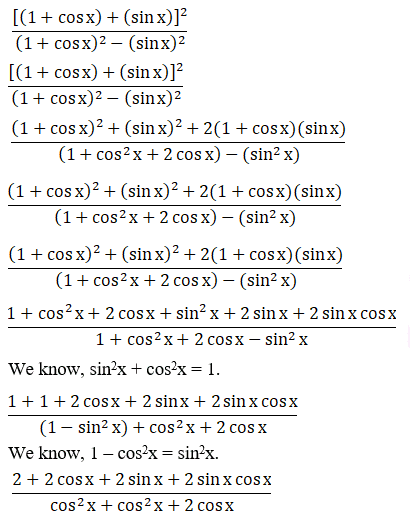
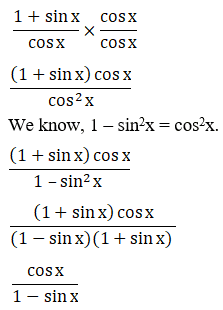
= LHS

∴ LHS = RHS

Hence Proved.

10.

Solution:

Let us consider LHS:

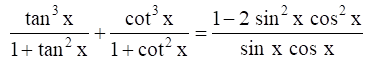
By using the formulas,

1 + tan²x = sec²x and 1 + cot²x = cosec²x

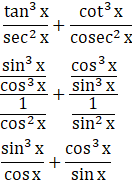
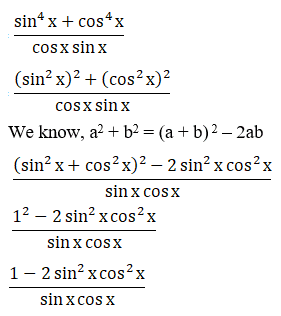
= RHS

∴ LHS = RHS

Hence Proved.

11

Solution:

Let us consider LHS:

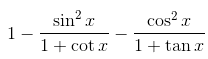
By using the formula,

tan θ = sin θ / cos θ;

cot θ = cos θ / sin θ

Now,

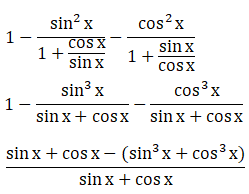
By using the formula, a³ + b³ = (a + b) (a² + b²– ab)

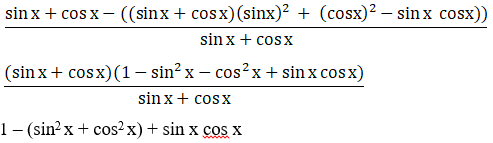
We know, sin² x + cos² x = 1.

1 – 1 + sin x cos x

Sin x cos x

= RHS

∴ LHS = RHS

Hence proved.

12. 

Solution:

Let us consider LHS:

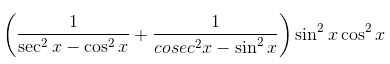
By using the formula,

cosec θ = 1/sin θ,

sec θ = 1/cos θ;

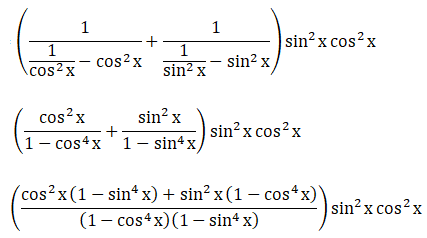
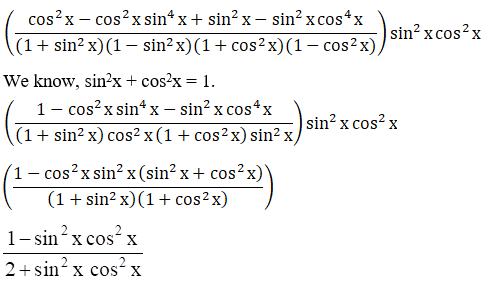
= RHS

∴ LHS = RHS

Hence proved.

13. (1 + tan α tan β) ² + (tan α – tan β) ² = sec² α sec² β

Solution:

Let us consider LHS: (1 + tan α tan β) ² + (tan α – tan β) ²

1+ tan² α tan² β + 2 tan α tan β + tan² α + tan² β – 2 tan α tan β

1 + tan² α tan² β + tan² α + tan² β

tan² α (tan² β + 1) + 1 (1 + tan² β)

(1 + tan² β) (1 + tan² α)

We know, 1 + tan² θ = sec² θ

So,

sec² α sec² β

= RHS

∴ LHS = RHS

Hence proved.

EXERCISE 5.2 PAGE NO: 5.25

1. Find the values of the other five trigonometric functions in each of the following:

(i) cot x = 12/5, x in quadrant III

(ii) cos x = -1/2, x in quadrant II

(iii) tan x = 3/4, x in quadrant III

(iv) sin x = 3/5, x in quadrant I

Solution:

(i) cot x = 12/5, x in quadrant III

In third quadrant, tan x and cot x are positive. sin x, cos x, sec x, cosec x are negative.

By using the formulas,

tan x = 1/cot x

= 1/(12/5)

= 5/12

cosec x = –√(1 + cot² x)

= –√(1 + (12/5)²)

= –√(25+144)/25

= –√(169/25)

= -13/5

sin x = 1/cosec x

= 1/(-13/5)

= -5/13

cos x = – √(1 – sin² x)

= – √(1 – (-5/13)²)

= – √(169-25)/169

= – √(144/169)

= -12/13

sec x = 1/cos x

= 1/(-12/13)

= -13/12

∴ sin x = -5/13, cos x = -12/13, tan x = 5/12, cosec x = -13/5, sec x = -13/12

(ii) cos x = -1/2, x in quadrant II

In second quadrant, sin x and cosec x are positive. tan x, cot x, cos x, sec x are negative.

By using the formulas,

sin x = √(1 – cos² x)

= √(1 – (-1/2)²)

= √(4-1)/4

= √(3/4)

= √3/2

tan x = sin x/cos x

= (√3/2)/(-1/2)

= -√3

cot x = 1/tan x

= 1/-√3

= -1/√3

cosec x = 1/sin x

= 1/(√3/2)

= 2/√3

sec x = 1/cos x

= 1/(-1/2)

= -2

∴ sin x = √3/2, tan x = -√3, cosec x = 2/√3, cot x = -1/√3 sec x = -2

(iii) tan x = 3/4, x in quadrant III

In third quadrant, tan x and cot x are positive. sin x, cos x, sec x, cosec x are negative.

By using the formulas,

sin x = √(1 – cos² x)

= – √(1-(-4/5)²)

= – √(25-16)/25

= – √(9/25)

= – 3/5

cos x = 1/sec x

= 1/(-5/4)

= -4/5

cot x = 1/tan x

= 1/(3/4)

= 4/3

cosec x = 1/sin x

= 1/(-3/5)

= -5/3

sec x = -√(1 + tan² x)

= – √(1+(3/4)²)

= – √(16+9)/16

= – √ (25/16)

= -5/4

∴ sin x = -3/5, cos x = -4/5, cosec x = -5/3, sec x = -5/4, cot x = 4/3

(iv) sin x = 3/5, x in quadrant I

In first quadrant, all trigonometric ratios are positive.

So, by using the formulas,

tan x = sin x/cos x

= (3/5)/(4/5)

= 3/4

cosec x = 1/sin x

= 1/(3/5)

= 5/3

cos x = √(1-sin² x)

= √(1 – (-3/5)²)

= √(25-9)/25

= √(16/25)

= 4/5

sec x = 1/cos x

= 1/(4/5)

= 5/4

cot x = 1/tan x

= 1/(3/4)

= 4/3

∴ cos x = 4/5, tan x = 3/4, cosec x = 5/3, sec x = 5/4, cot x = 4/3

2. If sin x = 12/13 and lies in the second quadrant, find the value of sec x + tan x.

Solution:

Given:

Sin x = 12/13 and x lies in the second quadrant.

We know, in second quadrant, sin x and cosec x are positive and all other ratios are negative.

By using the formulas,

Cos x = √(1-sin² x)

= – √(1-(12/13)²)

= – √(1- (144/169))

= – √(169-144)/169

= -√(25/169)

= – 5/13

We know,

tan x = sin x/cos x

sec x = 1/cos x

Now,

tan x = (12/13)/(-5/13)

= -12/5

sec x = 1/(-5/13)

= -13/5

Sec x + tan x = -13/5 + (-12/5)

= (-13-12)/5

= -25/5

= -5

∴ Sec x + tan x = -5

3. If sin x = 3/5, tan y = 1/2 and π/2 < x< π< y< 3π/2 find the value of 8 tan x -√5 sec y.

Solution:

Given:

sin x = 3/5, tan y = 1/2 and π/2 < x< π< y< 3π/2

We know that, x is in second quadrant and y is in third quadrant.

In second quadrant, cos x and tan x are negative.

In third quadrant, sec y is negative.

By using the formula,

cos x = – √(1-sin² x)

tan x = sin x/cos x

Now,

cos x = – √(1-sin² x)

= – √(1 – (3/5)²)

= – √(1 – 9/25)

= – √((25-9)/25)

= – √(16/25)

= – 4/5

tan x = sin x/cos x

= (3/5)/(-4/5)

= 3/5 × -5/4

= -3/4

We know that sec y = – √(1+tan² y)

= – √(1 + (1/2)²)

= – √(1 + 1/4)

= – √((4+1)/4)

= – √(5/4)

= – √5/2

Now, 8 tan x – √5 sec y = 8(-3/4) – √5(-√5/2)

= -6 + 5/2

= (-12+5)/2

= -7/2

∴ 8 tan x – √5 sec y = -7/2

4. If sin x + cos x = 0 and x lies in the fourth quadrant, find sin x and cos x.

Solution:

Given:

Sin x + cos x = 0 and x lies in fourth quadrant.

Sin x = -cos x

Sin x/cos x = -1

So, tan x = -1 (since, tan x = sin x/cos x)

We know that, in fourth quadrant, cos x and sec x are positive and all other ratios are negative.

By using the formulas,

Sec x = √(1 + tan² x)

Cos x = 1/sec x

Sin x = – √(1- cos² x)

Now,

Sec x = √(1 + tan² x)

= √(1 + (-1)²)

= √2

Cos x = 1/sec x

= 1/√2

Sin x = – √(1 – cos² x)

= – √(1 – (1/√2)²)

= – √(1 – (1/2))

= – √((2-1)/2)

= – √(1/2)

= -1/√2

∴ sin x = -1/√2 and cos x = 1/√2

5. If cos x = -3/5 and π<x<3π/2 find the values of other five trigonometric functions and hence evaluate 

Solution:

Given:

cos x= -3/5 and π <x < 3π/2

We know that in the third quadrant, tan x and cot x are positive and all other rations are negative.

By using the formulas,

Sin x = – √(1-cos² x)

Tan x = sin x/cos x

Cot x = 1/tan x

Sec x = 1/cos x

Cosec x = 1/sin x

Now,

Sin x = – √(1-cos² x)

= – √(1-(-3/5)²)

= – √(1-9/25)

= – √((25-9)/25)

= – √(16/25)

= – 4/5

Tan x = sin x/cos x

= (-4/5)/(-3/5)

= -4/5 × -5/3

= 4/3

Cot x = 1/tan x

= 1/(4/3)

= 3/4

Sec x = 1/cos x

= 1/(-3/5)

= -5/3

Cosec x = 1/sin x

= 1/(-4/5)

= -5/4

∴ 
= [(-5/4) + (3/4)] / [(-5/3) – (4/3)]

= [(-5+3)/4] / [(-5-4)/3]

= [-2/4] / [-9/3]

= [-1/2] / [-3]

= 1/6

EXERCISE 5.3 PAGE NO: 5.39

1. Find the values of the following trigonometric ratios:

(i) sin 5π/3

(ii) sin 17π

(iii) tan 11π/6

(iv) cos (-25π/4)

(v) tan 7π/4

(vi) sin 17π/6

(vii) cos 19π/6

(viii) sin (-11π/6)

(ix) cosec (-20π/3)

(x) tan (-13π/4)

(xi) cos 19π/4

(xii) sin 41π/4

(xiii) cos 39π/4

(xiv) sin 151π/6

Solution:

(i) sin 5π/3

5π/3 = (5π/3 × 180)^o

= 300^o

= (90×3 + 30)^o

Since, 300^o lies in IV quadrant in which sine function is negative.

sin 5π/3 = sin (300)^o

= sin (90×3 + 30)^o

= – cos 30^o

= – √3/2

(ii) sin 17π

Sin 17π = sin 3060^o

= sin (90×34 + 0)^o

Since, 3060^o lies in the negative direction of x-axis i.e., on boundary line of II and III quadrants.

Sin 17π = sin (90×34 + 0)^o

= – sin 0^o

= 0

(iii) tan 11π/6

tan 11π/6 = (11/6 × 180)^o

= 330^o

Since, 330^o lies in the IV quadrant in which tangent function is negative.

tan 11π/6 = tan (300)^o

= tan (90×3 + 60)^o

= – cot 60^o

= – 1/√3

(iv) cos (-25π/4)

cos (-25π/4) = cos (-1125)^o

= cos (1125)^o

Since, 1125^o lies in the I quadrant in which cosine function is positive.

cos (1125)^o = cos (90×12 + 45)^o

= cos 45^o

= 1/√2

(v) tan 7π/4

tan 7π/4 = tan 315^o

= tan (90×3 + 45)^o

Since, 315^o lies in the IV quadrant in which tangent function is negative.

tan 315^o = tan (90×3 + 45)^o

= – cot 45^o

= -1

(vi) sin 17π/6

sin 17π/6 = sin 510^o

= sin (90×5 + 60)^o

Since, 510^o lies in the II quadrant in which sine function is positive.

sin 510^o = sin (90×5 + 60)^o

= cos 60^o

= 1/2

(vii) cos 19π/6

cos 19π/6 = cos 570^o

= cos (90×6 + 30)^o

Since, 570^o lies in III quadrant in which cosine function is negative.

cos 570^o = cos (90×6 + 30)^o

= – cos 30^o

= – √3/2

(viii) sin (-11π/6)

sin (-11π/6) = sin (-330^o)

= – sin (90×3 + 60)^o

Since, 330^o lies in the IV quadrant in which the sine function is negative.

sin (-330^o) = – sin (90×3 + 60)^o

= – (-cos 60^o)

= – (-1/2)

= 1/2

(ix) cosec (-20π/3)

cosec (-20π/3) = cosec (-1200)^o

= – cosec (1200)^o

= – cosec (90×13 + 30)^o

Since, 1200^o lies in the II quadrant in which cosec function is positive.

cosec (-1200)^o = – cosec (90×13 + 30)^o

= – sec 30^o

= -2/√3

(x) tan (-13π/4)

tan (-13π/4) = tan (-585)^o

= – tan (90×6 + 45)^o

Since, 585^o lies in the III quadrant in which the tangent function is positive.

tan (-585)^o = – tan (90×6 + 45)^o

= – tan 45^o

= -1

(xi) cos 19π/4

cos 19π/4 = cos 855^o

= cos (90×9 + 45)^o

Since, 855^o lies in the II quadrant in which the cosine function is negative.

cos 855^o = cos (90×9 + 45)^o

= – sin 45^o

= – 1/√2

(xii) sin 41π/4

sin 41π/4 = sin 1845^o

= sin (90×20 + 45)^o

Since, 1845^o lies in the I quadrant in which the sine function is positive.

sin 1845^o = sin (90×20 + 45)^o

= sin 45^o

= 1/√2

(xiii) cos 39π/4

cos 39π/4 = cos 1755^o

= cos (90×19 + 45)^o

Since, 1755^o lies in the IV quadrant in which the cosine function is positive.

cos 1755^o = cos (90×19 + 45)^o

= sin 45^o

= 1/√2

(xiv) sin 151π/6

sin 151π/6 = sin 4530^o

= sin (90×50 + 30)^o

Since, 4530^o lies in the III quadrant in which the sine function is negative.

sin 4530^o = sin (90×50 + 30)^o

= – sin 30^o

= -1/2

2. prove that:
(i) tan 225^o cot 405^o + tan 765^o cot 675^o = 0

(ii) sin 8π/3 cos 23π/6 + cos 13π/3 sin 35π/6 = 1/2

(iii) cos 24^o + cos 55^o + cos 125^o + cos 204^o + cos 300^o = 1/2

(iv) tan (-125^o) cot (-405^o) – tan (-765^o) cot (675^o) = 0

(v) cos 570^o sin 510^o + sin (-330^o) cos (-390^o) = 0

(vi) tan 11π/3 – 2 sin 4π/6 – 3/4 cosec² π/4 + 4 cos² 17π/6 = (3 – 4√3)/2

(vii) 3 sin π/6 sec π/3 – 4 sin 5π/6 cot π/4 = 1

Solution:

(i) tan 225^o cot 405^o + tan 765^o cot 675^o = 0

Let us consider LHS:

tan 225° cot 405° + tan 765° cot 675°

tan (90° × 2 + 45°) cot (90° × 4 + 45°) + tan (90° × 8 + 45°) cot (90° × 7 + 45°)

We know that when n is odd, cot → tan.

tan 45° cot 45° + tan 45° [-tan 45°]

tan 45° cot 45° – tan 45° tan 45°

1 × 1 – 1 × 1

1 – 1

0 = RHS

∴ LHS = RHS

Hence proved.

(ii) sin 8π/3 cos 23π/6 + cos 13π/3 sin 35π/6 = 1/2

Let us consider LHS:

sin 8π/3 cos 23π/6 + cos 13π/3 sin 35π/6

sin 480° cos 690° + cos 780° sin 1050°

sin (90° × 5 + 30°) cos (90° × 7 + 60°) + cos (90° × 8 + 60°) sin (90° × 11 + 60°)

We know that when n is odd, sin → cos and cos → sin.

cos 30° sin 60° + cos 60° [-cos 60°]

√3/2 × √3/2 – 1/2 × 1/2

3/4 – 1/4

2/4

1/2

= RHS

∴ LHS = RHS

Hence proved.

(iii) cos 24^o + cos 55^o + cos 125^o + cos 204^o + cos 300^o = 1/2

Let us consider LHS:

cos 24^o + cos 55^o + cos 125^o + cos 204^o + cos 300^o

cos 24° + cos (90° × 1 – 35°) + cos (90° × 1 + 35°) + cos (90° × 2 + 24°) + cos (90° × 3 + 30°)

We know that when n is odd, cos → sin.

cos 24° + sin 35° – sin 35° – cos 24° + sin 30°

0 + 0 + 1/2

1/2

= RHS

∴ LHS = RHS

Hence proved.

(iv) tan (-125^o) cot (-405^o) – tan (-765^o) cot (675^o) = 0

Let us consider LHS:

tan (-125^o) cot (-405^o) – tan (-765^o) cot (675^o)

We know that tan (-x) = -tan (x) and cot (-x) = -cot (x).

[-tan (225°)] [-cot (405°)] – [-tan (765°)] cot (675°)

 

tan (225°) cot (405°) + tan (765°) cot (675°)

tan (90° × 2 + 45°) cot (90° × 4 + 45°) + tan (90° × 8 + 45°) cot (90° × 7 + 45°)

tan 45° cot 45° + tan 45° [-tan 45°]

1 × 1 + 1 × (-1)

1 – 1

0

= RHS

∴ LHS = RHS

Hence proved.

(v) cos 570^o sin 510^o + sin (-330^o) cos (-390^o) = 0

Let us consider LHS:

cos 570^o sin 510^o + sin (-330^o) cos (-390^o)

We know that sin (-x) = -sin (x) and cos (-x) = +cos (x).

cos 570^o sin 510^o + [-sin (330^o)] cos (390^o)

cos 570^o sin 510^o – sin (330^o) cos (390^o)

cos (90° × 6 + 30°) sin (90° × 5 + 60°) – sin (90° × 3 + 60°) cos (90° × 4 + 30°)

We know that cos is negative at 90° + θ i.e. in Q₂ and when n is odd, sin → cos and cos → sin.

-cos 30° cos 60° – [-cos 60°] cos 30°

-cos 30° cos 60° + cos 60° cos 30°

0

= RHS

∴ LHS = RHS

Hence proved.

(vi) tan 11π/3 – 2 sin 4π/6 – 3/4 cosec² π/4 + 4 cos² 17π/6 = (3 – 4√3)/2

Let us consider LHS:

tan 11π/3 – 2 sin 4π/6 – 3/4 cosec² π/4 + 4 cos² 17π/6

tan (11 × 180^o)/3 – 2 sin (4 × 180^o)/6 – 3/4 cosec² 180^o/4 + 4 cos² (17 × 180^o)/6

tan 660^o – 2 sin 120^o – 3/4 (cosec 45^o)² + 4 (cos 510^o)²

tan (90° × 7 + 30°) – 2 sin (90° × 1 + 30°) – 3/4 [cosec 45°]² + 4 [cos (90° × 5 + 60°)]²

We know that tan and cos is negative at 90° + θ i.e. in Q₂ and when n is odd, tan → cot, sin → cos and cos → sin.

[-cot 30°] – 2 cos 30° – 3/4 [cosec 45°]² + [-sin 60°]²

 

– cot 30° – 2 cos 30° – 3/4 [cosec 45°]² + [sin 60°]²

-√3 – 2√3/2 – 3/4 (√2)² + 4 (√3/2)²

-√3 – √3 – 6/4 + 12/4

(3 – 4√3)/2

= RHS

∴ LHS = RHS

Hence proved.

(vii) 3 sin π/6 sec π/3 – 4 sin 5π/6 cot π/4 = 1

Let us consider LHS:

3 sin π/6 sec π/3 – 4 sin 5π/6 cot π/4

3 sin 180^o/6 sec 180^o/3 – 4 sin 5(180^o)/6 cot 180^o/4

3 sin 30° sec 60° – 4 sin 150° cot 45°

3 sin 30° sec 60° – 4 sin (90° × 1 + 60°) cot 45°

We know that when n is odd, sin → cos.

3 sin 30° sec 60° – 4 cos 60° cot 45°

3 (1/2) (2) – 4 (1/2) (1)

3 – 2

1

= RHS

∴ LHS = RHS

Hence proved.

3. Prove that:

(i)

(ii)

 

(iii)

(iv)

(v)

Solution:

(i) 

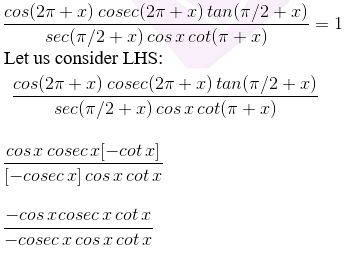
1 = RHS

∴ LHS = RHS

Hence proved.

(ii)

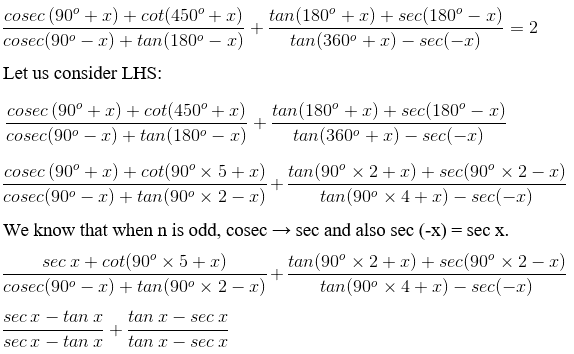
1 + 1

2 = RHS

∴ LHS = RHS

Hence proved.

(iii)

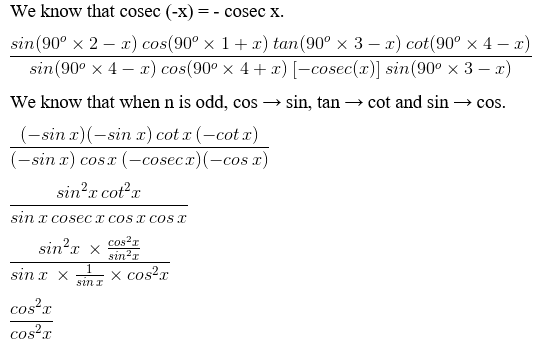
1 = RHS

∴ LHS = RHS

Hence proved.

(iv)

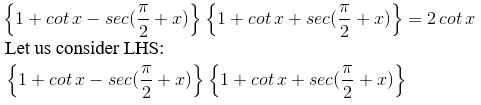
{1 + cot x – (-cosec x)} {1 + cot x + (-cosec x)}

{1 + cot x + cosec x} {1 + cot x – cosec x}

{(1 + cot x) + (cosec x)} {(1 + cot x) – (cosec x)}

By using the formula, (a + b) (a – b) = a² – b²

(1 + cot x)² – (cosec x)²

1 + cot² x + 2 cot x – cosec² x

We know that 1 + cot² x = cosec² x

cosec² x + 2 cot x – cosec² x

2 cot x = RHS

∴ LHS = RHS

Hence proved.

(v)

1 = RHS

∴ LHS = RHS

Hence proved.

4. Prove that: sin² π/18 + sin² π/9 + sin² 7π/18 + sin² 4π/9 = 2

Solution:

Let us consider LHS:

sin² π/18 + sin² π/9 + sin² 7π/18 + sin² 4π/9

sin² π/18 + sin² 2π/18 + sin² 7π/18 + sin² 8π/18

sin² π/18 + sin² 2π/18 + sin² (π/2 – 2π/18) + sin² (π/2 – π/18)

We know that when n is odd, sin → cos.

sin² π/18 + sin² 2π/18 + cos² 2π/18 + cos² 2π/18

when rearranged,

sin² π/18 + cos² 2π/18 + sin² π/18 + cos² 2π/18

We know that sin² + cos²x = 1.

So,

1 + 1

2 = RHS

∴ LHS = RHS

Hence proved.

Important Topics Class 11 Maths Chapter 23

RD Sharma Solutions Class 11 Maths Chapter 5, undoubtedly is one of the chapters which students need to take seriously from the very beginning of the course curriculum. So, to make it better, have a quick look at the important topics that are there, that needs to be discussed several times before appearing for the exam. Besides helping you with scoring well, these RD Sharma Solution will let you enhance your exam performance.

• Trigonometric functions of a real number.
• Values of trigonometric functions.
• Trigonometric identities.
• Fundamental trigonometric identities.
• Signs of trigonometric functions.
• Variations in values of trigonometric functions in different quadrants.
• Values of trigonometric functions at allied angles.
• Periodic functions.
• Even and odd functions.

Access Other Important Chapters of RD Sharma Solutions Class 11 Maths 

• Chapter 1 – Sets
• Chapter 2 – Relations
• Chapter 3 – Functions
• Chapter 4 – Measurement of Angles
• Chapter 6 – Graphs of Trigonometric Functions
• Chapter 7 – Trigonometric Ratios of Compound Angles
• Chapter 8 – Transformation Formulae
• Chapter 9 – Trigonometric Ratios of Multiple and Sub Multiple Angles
• Chapter 10 – Sine and Cosine Formulae and Their Applications
• Chapter 11 – Trigonometric Equations

Hence paying focus in this chapter is what you need to do right now. Have your copy of RD Sharma Solutions CBSE Class 11 Maths Chapter 5 PDF and begin your exam preparation.

FAQs on RD Sharma Solutions Class 11 Maths Chapter 5